An active-learning lesson that targets student understanding of population growth in ecology
Published online:
Abstract
Effective teaching and learning of population ecology requires integration of quantitative literacy skills. To facilitate student learning in population ecology and provide students with the opportunity to develop and apply quantitative skills, we designed a clicker-based lesson in which students investigate how ecologists measure and model population size. This lesson asks students to "engage like scientists" as they make predictions, plot data, perform calculations, and evaluate evidence. The lesson was taught in three sections of a large enrollment undergraduate class and assessed using a pre/post-test, in-class clicker-based questions, and multiple-choice exam questions. Student performance increased following peer discussion of clicker questions and on post-test questions. Students also performed well on the end-of-unit exam questions.
Citation
Trenckmann, E., Smith, M.K., Pelletreau, K.N., and Summers, M.M. 2017. An active-learning lesson that targets student understanding of population growth in ecology. CourseSource. https://doi.org/10.24918/cs.2017.11Society Learning Goals
Ecology
- Populations
- How do populations change over time?
Lesson Learning Goals
- How do populations change over time?
- Students will know that many different growth curves and patterns exist for organisms in nature. They will understand that modeling helps to describe and predict population growth over time.
Lesson Learning Objectives
Students will be able to:- Calculate and compare population density and abundance.
- Identify whether a growth curve describes exponential, linear, and/or logistic growth.
- Describe and calculate a population's growth rate using linear, exponential, and logistic models.
- Explain the influence of carrying capacity and population density on growth rate.
Article Context
Course
Article Type
Course Level
Bloom's Cognitive Level
Vision and Change Core Competencies
Vision and Change Core Concepts
Class Type
Class Size
Audience
Lesson Length
Pedagogical Approaches
Principles of How People Learn
Assessment Type
INTRODUCTION
Quantitative reasoning and literacy skills are essential for many careers, particularly those in biology, where individuals encounter a diversity of challenges that require application and integration of approaches (1,2). Biology education researchers have advocated integrating quantitative skills with biology content to prepare students to address such challenges, which span sectors including health, education, the environment, and complex social issues (3,4,5). Incorporating quantitative reasoning into lessons where students also develop science process skills (e.g., posing questions, analyzing and interpreting evidence, developing models, and generating testable predictions) encourages interdisciplinary thinking and problem-solving (1,6). However, previous studies have revealed that students have difficulties transferring skills between math and science courses. Undergraduate biology students struggle with higher-order quantitative thinking (e.g., analyzing, evaluating, and drawing conclusions) in biological contexts, for example, using raw data to generate graphs (3,7); interpreting bar graphs and scatterplots (3); understanding independent and dependent variables (7); summarizing trends from data with variation (7,8); and articulating data driven arguments (3). Introductory biology students also have difficulty performing simple calculations (such as calculating a mean) and representing calculations graphically (3).
Population ecology is a topic well suited for the development of quantitative reasoning and literacy skills. At both the introductory and advanced levels, students are asked to model population growth using graphs, measure population size, and estimate carrying capacity (9,10). However, students often struggle with integrating quantitative skills with conceptual understanding and practical application (11,12). For example, undergraduate students have incorrectly predicted that a population would likely exceed carrying capacity if a non-limiting factor increased (11) and that all population sizes will level off regardless of the resources available (13). They have also incorrectly explained that competition only occurs between organisms of the same species (11,12). Although instructional tools currently available target some of these persistent conceptual difficulties (14-19), there is a need for materials that have been explicitly designed to investigate student thinking and learning progressions. In addition, because the available instructional materials on this topic are largely computer models and lab based activities, there is a need for tools that integrate quantitative skills in a lecture format.
Here we describe an interactive in-class lesson that targets conceptual difficulties in population ecology and seeks to develop students' quantitative reasoning. This 50-minute clicker-based lesson focuses on exploring population growth in a barnacle population using authentic data from a seminal study in ecology (20). Throughout the lesson, students predict, plot, calculate, and interpret data to learn the methods used by ecologists to measure, describe, and model population growth.
INTENDED AUDIENCE
This lesson is intended for undergraduate introductory biology courses. It was given to students in a large enrollment Introductory Biology course for majors and non-majors at the University of Maine (n=766; students divided into three class sections). In this class, the mean SAT Math score was 525 (range from 320-770; 87% of students took the SAT) and mean ACT Math score was 23 (range 14-33; 18% of students took the ACT).
REQUIRED LEARNING TIME
This lesson was designed for a 50-minute class period.
PRE-REQUISITE STUDENT KNOWLEDGE
Before this lesson, students participated in a lecture with clicker questions that was focused on the following topics: abiotic and biotic factors responsible for determining population size, the definitions of carrying capacity and regulation, and different examples of populations that exhibit logistic and exponential growth. They were also asked to complete an assigned textbook reading (9) that introduced population ecology and an overview of biotic and abiotic factors, density, exponential population growth, logistic and exponential growth curves and equations, carrying capacity, and density-dependent regulation.
PRE-REQUISITE TEACHER KNOWLEDGE
We recommend that instructors are familiar with how ecologists study population growth and the main concepts covered in the lesson (measurements of population size; the equations that describe linear, exponential, and logistic growth; the role of carrying capacity; and examples of different growth models and their applications). Instructors will also benefit from basic knowledge about barnacles and the intertidal zone. Information about each concept, barnacles, and the intertidal zone are included in the instructor notes within the lesson slides (Supporting File S1: Lesson Presentation Slides with Instructor Notes). Recommended population ecology resources are also provided in Supporting File S2: Instructor Population Ecology Resources.
We also suggest that instructors familiarize themselves with common and persistent conceptual difficulties related to population growth. We used the EcoEvo-MAPs assessment tool (11) at the beginning of this course to identify some of the ecology and evolution concepts with which our students struggle.
SCIENTIFIC TEACHING THEMES
ACTIVE LEARNING
Students are actively engaged in their learning throughout the lesson. Students make predictions, answer clicker questions, engage in peer discussion, participate in group problem-solving, answer questions in their own words on a worksheet, and respond to instructor questions. The instructor (author KP) who taught this lesson was observed using the Classroom Observation Protocol for Undergraduate STEM (COPUS) (21). This observation protocol uses a series of codes to characterize instructor and student behavior in the classroom and documents those behaviors in two-minute intervals throughout the duration of the lesson (21,22). The summary of COPUS results (Figure 1) highlights the diversity of student-centered instructional practices and student participation in this lesson. For example, throughout the lesson more than 25% of the codes were "students talking to the class" (asking and answering questions) and more than 25% of the codes were "students working" (working individually, answering clicker questions, and working in groups). Similarly, for the instructor, nearly 75% of the codes were "guiding" (posing questions to students, asking clicker questions, following up with students, and moving and guiding).
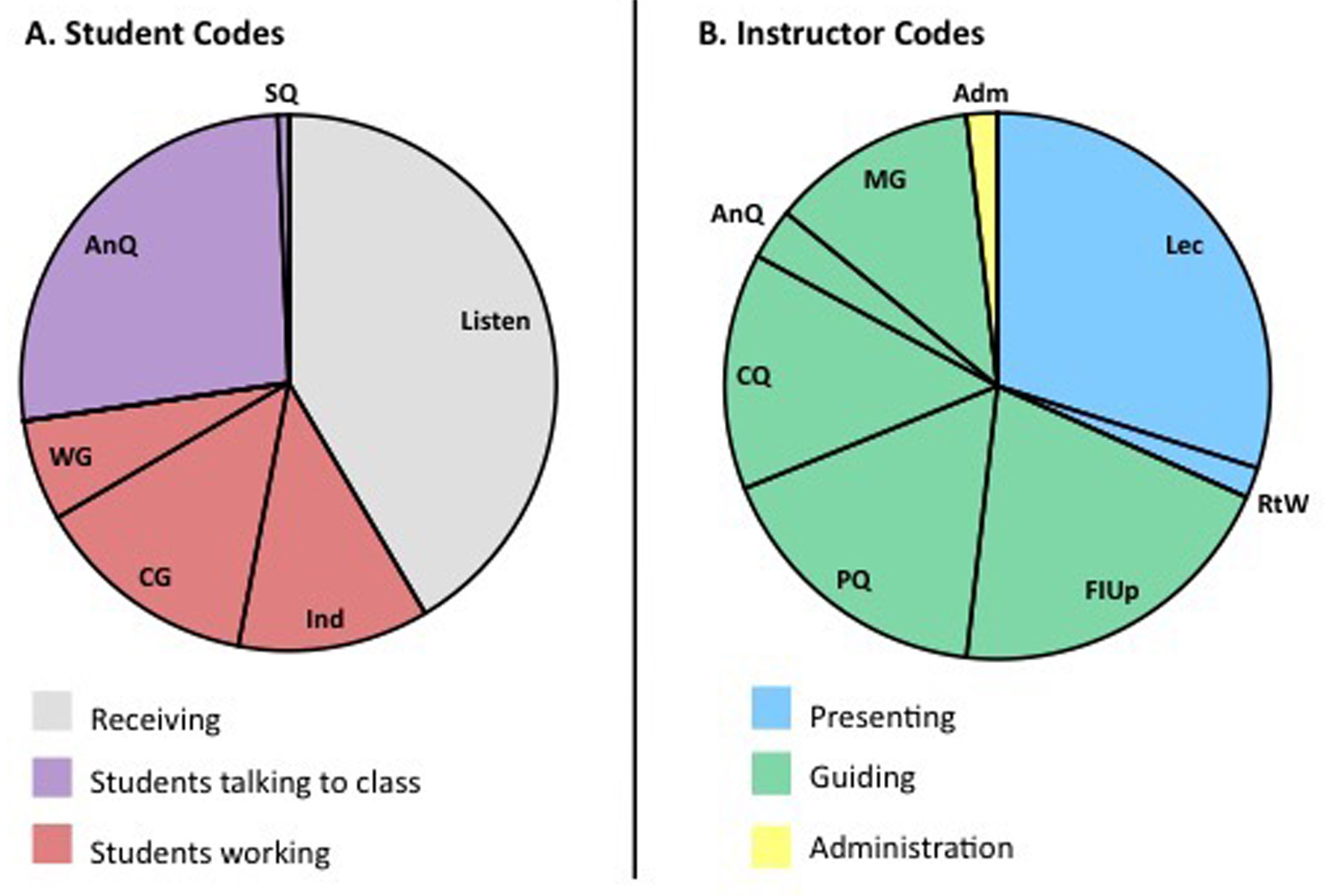
Figure 1. Average collapsed Classroom Observation Protocol for Undergraduate STEM (COPUS) (21, 22) codes from three sections of a University of Maine introductory biology course in which this lesson was used. Collapsed student codes: Receiving (Listen- Listening), Students Working (Ind- Individual Work, CQ- Clicker Question Discussion, WG- Worksheet Group Work), Students Talking to Class (AnQ- Answering Question, SQ- Student Question). Collapsed instructor codes: Presenting (Lec- Lecturing, RtW- Real Time Writing), Guiding (FlUp-Follow-up, PQ- Posing Questions, CQ-Clicker Question, AQ-Answer Question, MG-Moving and Guiding), Administration (Adm- Administration).
ASSESSMENT
Students were assessed using formative and summative questions aligned with the learning outcomes: quantifying population size, plotting and predicting population growth, calculating growth rate, and incorporating carrying capacity in logistic growth curves. We used clicker-based questions for formative, real-time assessment of student understanding during the lesson. Student responses are included in the lesson plan and in Supporting File 3: Clicker Questions and Student Responses. Summative assessment of student understanding included a pre/post-test and exam questions. We administered the pre/post-test on-line and each contained ten multiple-choice questions. Students were required to complete the pre-test in the 24-hours preceding the lesson. Students were given five days to complete the post-test, starting five days after the lesson. Pre/post-test questions are included in Supporting File S4: Pre/Post-Test Questions and Student Responses. Students also answered three multiple-choice exam questions three days after the lesson. Multiple-choice exam questions are provided in Supporting File S5: Exam Questions and Student Responses.
INCLUSIVE TEACHING
This lesson seeks to create a learning environment where students' academic, social, and cultural backgrounds can be an asset to their learning. We fostered this inclusive teaching environment through incorporating a variety of different teaching methods to meet the needs of students with diverse learning preferences, abilities, and backgrounds (23,24). For example, the concepts were presented and available to students on projected slides, the student worksheet, and through instructor and peer-led discussion. When questions were presented, students were given the opportunity to first think and write on their own, then discuss in small groups, and finally report out to the entire class. In addition, we used an anonymous response system (clickers) with peer discussion to reduce student discomfort (25) and promote a collaborative learning environment (26-28)
LESSON PLAN
This lesson is designed for a 50-minute lecture and is intended to introduce students to quantitative skills used in population ecology. It can follow an introduction to population ecology provided either in a previous class session or as a pre-class assignment. Table 1 provides the progression of the clicker-based lesson with estimated timing.
PRE-CLASS PREPARATION
We recommend that students are familiar with and able to recall the following terms before the lesson: density; abundance; carrying capacity; density dependence; and linear, exponential, and logistic growth (Supporting File S2: Instructor Population Ecology Resources). This preparation can be achieved through prior readings, homework assignments, or lectures.
Instructors will need to facilitate conversations among students during this lesson. Additional instructional resources on the content and quantitative skills covered are provided in Supporting File S2: Instructor Population Ecology Resources.
THINK-PAIR-SHARE AND USE OF CLICKERS
Think-Pair-Share (TPS) is a classroom-based active learning strategy in which students think about a problem posed by an instructor individually, work in pairs to solve the problem, and finally share their ideas with the entire class (29). This model allows students to think individually about the questions posed, reflect on their own thinking, and obtain immediate feedback from their peers and instructor (30). During the in-class lesson, students answered nine questions using the TPS model. Clickers were used to facilitate TPS. Students first answered individually ("think") followed by discussion with a neighbor and revote ("pair"). After the revote, the instructor asked for students to volunteer their thinking ("share") and then discussed the correct answer and student thinking. This combination of peer and instructor-led discussion has been shown to result in greater student gains than either peer discussion or instructor explanation alone (27). In the instructor slides and student worksheet, each classroom-discussion open-response TPS question opportunity is identified by "TPSQ" and each clicker question TPS opportunity is abbreviated "CQ" (Supporting File S1: Lesson Presentation Slides with Instructor Notes, and Supporting File S6: Student Worksheet). Specific advice on the administration of each of these questions is given in the "Progressing Through the Lesson" section of this article and in the notes section of the lesson slides.
PROGRESSING THROUGH THE LESSON
1. INTRODUCTION AND ASSESSING PRIOR KNOWLEDGE (~10 MINUTES)
The lesson begins with an overview of population dynamics and engaging students' prior knowledge about barnacles. The instructor first defines population dynamics and informs students that they will be learning some of the methodology used by population ecologists: collecting and analyzing descriptive data and generating and evaluating mathematical models (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 2). The instructor describes modeling and then explains that although ecologists in general seek to understand how all organisms in an ecosystem interact, population ecologists approach this goal by focusing on individual populations that they can observe and manipulate with experiments (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 3). The instructor can also introduce the seminal role that barnacles played in developing the field and methodology of population ecology, and why barnacles are a good system for studying population growth (20). Since most of the lesson focuses on barnacle population growth, the instructor next gives students the opportunity to engage their prior knowledge about barnacle biology and what affects their population size.
TPSQ1. What affects barnacle population size?
For approximately four minutes, students brainstorm in small groups and write (on their worksheet) the factors that they think might affect barnacle population size (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 4). This time allows students to review and ask questions about barnacles before seeing data related to barnacle population growth. In our introductory biology course students mentioned: temperature, salinity, human disturbance (crushing), competition, food availability, exposure to the air, wave action, predation, and disease as likely factors affecting barnacle population size. Typical questions that arose about barnacles included, "How long can barnacles live outside of the water? (answer: up to about 6 weeks); "Do barnacles reproduce sexually?" (answer: yes, most species of barnacles are hermaphroditic); "What type of animal are barnacles" (answer: Arthropoda, Crustacea) and "What preys on barnacles?" (answer: fish, limpets, crabs, sea stars, and marine snails such as whelks).
Following small group discussion, the instructor can solicit answers from students. If desired, the instructor can write these answers on a board and identify biotic versus abiotic factors. A summary slide of factors that students mentioned in the lesson is provided (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 5). To transition to the next section of the lecture, the instructor focuses students' attention to the variable of interest on the summary slide - the number of barnacles.
2. QUANTIFYING POPULATION SIZE (~5 MINUTES)
This section of the lesson focuses on how ecologists measure and describe population size. The instructor first introduces density and abundance, explaining that the choice of using density or abundance depends on the organism and study design (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 6). For example, when observing a population of elephants in the savannah, abundance is likely a more useful measure of population size because you can easily count every individual. If, however, bacteria in a flask are being observed, density is a more appropriate measurement due to the constraints of counting every single individual. Since having a clear understanding of these two measurements is important, the instructor next gives students the opportunity to compare and calculate density and abundance.
CQ1. Which of the following statements describes the two study sites below?
Students observe a diagram and compare the density and abundance of barnacles. Using their clicker, they select from the following answer choices: A) site two has a greater abundance and density than site one; B) site one has a lower abundance but equal density to site two; C) site one has a lower abundance but greater density than site two; or D) the density and abundance are equal for both sites (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slides 7-8). After responding to the question individually, students discuss in small groups and revote. During this time, the instructor should encourage students to discuss their reasoning and how they calculated density and abundance. In our class, 70% of students answered correctly before peer discussion (answer C) and 93% were correct following peer discussion (Supporting File S3: Clicker Questions and Student Responses). These results suggest that students had a good understanding of density and abundance after receiving the definition of both.
After small group discussion, the instructor can ask students to describe how they arrived at their answer. In our class, students described two different ways of determining the correct answer. Some students calculated the density while others used the diagram to visually compare the density and abundance of barnacles in each study site. After restating different problem-solving techniques, the instructor provides an explanation of how to calculate density and abundance using mathematical equations (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slides 9-10).
3. PREDICTING AND PLOTTING POPULATION GROWTH (~10 MINUTES)
Students are asked to make a prediction using a graph and then plot data of population growth over time. The instructor first introduces the methodology used by Connell, 1961 (20) to study barnacle population growth in the intertidal zone (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 11). The slide includes a diagram of the experimental set up to illustrate that the number of barnacles increases over time. Emphasis on the following experimental features is important for this lesson: 1) the author measured the density of the population (y-axis); 2) the study was conducted over two months (x-axis); and 3) cages were used to prevent predation during the experiment (20).
To provide students with the opportunity to think about the x-and y-axes on the graph, and also how barnacle populations might grow over time, the instructor next asks students to predict barnacle population size over the course of the experiment (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 12). .
TPSQ2. Draw on your paper what you would expect the growth of this population to look like over the 30-day experiment.
CQ2. Select the growth curve that most resembles your prediction for barnacle population growth.
Students use the blank graph on their worksheet (with the y- and x-axis provided and labeled) to predict barnacle population size over the course of the study. To easily quantify student predictions, students answer a clicker-question (CQ2) where they individually vote for the growth curve - A) linear, B) exponential, or C) logistic - that most resembles their prediction (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 13). During the individual vote in the lesson, students chose all three growth curves (23% linear, 43% exponential, and 34% logistic). Students then talk with their peers and revote using their clickers. The instructor can encourage students to discuss their reasoning and what they think may influence the type of growth shown in the curve they selected. Following peer discussion in the lesson, 60% of students voted for the logistic growth curve, 7% selected linear, and 33% chose exponential.
After peer discussion, the instructor can solicit answers from students by having them raise their hands and share their initial prediction and reasoning. In the lesson, student reasoning for each of the growth curves included: (1) linear - "The population will increase steadily over time;" (2) exponential - "I don't know what the carrying capacity of the rock is, there is not enough information to know if or when the population will level off;" and (3) logistic - "Since the study was conducted on a single rock, I figured eventually the barnacles would run out of space, and there would be no more room for additional barnacles to settle, therefore the graph would level off." After discussing students' responses, the instructor provides students with the experimental data to plot and compare to their prediction.
TPSQ3. Use the data provided to plot the barnacle population growth.
CQ3. Select the growth curve that most resembles the curve you generated from the data provided.
Students use data from Connell, 1961 (20) to plot barnacle population density over time on their worksheet (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 14). To check that students are plotting the data correctly, students answer a clicker question (CQ3) where they select the growth curve - A) linear, B) exponential, or C) logistic - that most resembles their plot of the provided data (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 15-16). In our class, 99% of students answered correctly during the individual vote and the instructor skipped peer discussion. The instructor next says that while logistic growth is common, other mathematical models (e.g., linear and exponential) can be used to describe growth in populations. Also, since there are periods of linear and exponential growth in the barnacle data, all three of types of growth will be discussed further.
4. IDENTIFYING AND COMPARING GROWTH RATE (~8 MINUTES)
This part of the lesson targets the mathematical equations used to describe growth rate. The instructor first defines growth rate and then describes how it differs for the three growth curves (linear, exponential, and logistic), showing, but not yet explaining, the three mathematical equations (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 17). The instructor can emphasize that ecologists may be interested in determining how the growth rate changes, or might change, over time. The instructor then uses a clicker question to help students distinguish how growth rate differs in the three growth curves.
CQ4. The growth rate (or change in the population density) is:
Students use a diagram of the three different growth curves (linear, exponential, and logistic) and infer how the growth rate is changing over time in each. Using their clickers (CQ4), students select from the following answer choices: A) increasing over time in all three growth curves; B) increasing over time in the linear and exponential growth curves only; C) constant over time in all three growth curves; or D) constant in the linear growth curve and changes over time in the exponential and logistic growth curves (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slides 18-19). In our class, 74% of students answered correctly (D) before peer discussion, while 15% selected choice B. After peer discussion, the number of correct responses increased to 86%.
After small group discussion, the instructor solicits answers by having students raise their hands and explain how they arrived at their answer. In the lesson, student reasoning for selecting choice A included: "It looks like the growth rate is increasing in all three graphs, because the population size is getting larger over time." Reasoning for B included: "The population is increasing over time in the linear and exponential growth curves, however it is leveling off in the logistic." Reasoning for the correct answer D included: "If you look at the slope of the line, you will see that it is constant in the linear growth curve, however it changes over time in both the exponential and logistic growth curves."
Next, the instructor demonstrates how to calculate the growth rate at different time-points and population sizes using a linear and exponential growth model (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slides 20-22). The instructor first explains the variables used in the linear growth model: population size (N) and time (t), and introduces the technique of changing the values of the variables in the equation to see how the growth rate changes. By selecting two points on the linear growth curve, the instructor demonstrates to students how the growth rate is constant over time. This slide is also a good time to say that the slope of the line is the growth rate.
The instructor then introduces the variables used in the exponential growth model: population size (N), time (t), and the intrinsic rate of increase (r). The instructor describes the intrinsic rate of increase (r) as a value that ecologists estimate. The instructor explains that the simplest way to interpret r in the context of exponential growth is that if r=2/day, for every barnacle present, two additional barnacles will be added per day. The instructor can then use the technique of changing the values of the variables to see how the growth rate will vary. Here it is important that the instructor emphasizes that in exponential growth when the intrinsic rate of increase stays constant, as the population size increases, the growth rate increases.
5. DETERMINING THE INFLUENCE OF CARRYING CAPACITY (~10 MINUTES)
This section of the lesson addresses limitations to population growth. Using the Connell, 1961 data (20), the instructor provides an estimate of the carrying capacity (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 23). Here the instructor highlights that the first portion of the graph resembles exponential growth, but that there is a decrease in the growth rate as the barnacle population approaches ~80 barnacles per cm2, our estimate of carrying capacity (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 24). The instructor defines regulating mechanisms (those that influence population growth rate) and density-dependent regulation (which occurs when population growth rates are influenced by the density of the population). Here the instructor can ask students to volunteer likely regulating mechanisms for the barnacle population. In our class, students mentioned: food availability, predation, disease, and/or space. Given the experimental set-up, where food is plentiful and predation is prevented, the most likely regulating mechanism is space.
After discussing regulating mechanisms, the instructor shows students the logistic growth equation (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 25). First, the instructor notes that the beginning of the graph resembles exponential growth. The instructor then draws the connection that the first portion of the logistic equation is the same as the exponential growth equation (rN). Next, the instructor can ask students to identify the new variable in the second part of the equation (1-N/K) [answer: carrying capacity (K)]. The instructor can then request that students notice that the second part of the equation including K is (1-N/K).
CQ5. If r=2 per day and K=80 barnacles per cm2, how does increasing the population size (N) affect the population growth rate (dN/dt) in the logistic growth model?
Students now investigate why the equation is (1-N/K) and how the new variable (K) can result in a decreasing growth rate. They do so by calculating the growth rate for different population sizes (N), given values for the intrinsic growth rate (r) and carrying capacity (K). In this way, they determine what happens as the population approaches carrying capacity (i.e., as N increases) (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slides 26-28). Students work on these calculations in their small groups and select from the following (CQ5): A) as N approaches K, the growth rate increases; B) as N approaches K, the growth rate slows; or C) as N approaches K, the growth rate stays constant. If students are having difficulty, the instructor can provide an example calculation using a population size (N) of 40 (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 27). In our class, students struggled to use the logistic equation to calculate growth rate on their own, so the instructor allowed students to work together in pairs to answer the question (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 27). After the instructor-led example and group problem-solving, 76% of students correctly answered choice B. Students could have arrived at the correct answer to this question through reasoning or calculation.
After small group discussion, the instructor emphasizes how the equation models what happens to the growth rate as the population approaches carrying capacity (the growth rate increases initially, is fastest at half the carrying capacity, and decreases as the population continues to approach carrying capacity). Next, the instructor shows students the growth rates calculated for the Connell, 1961 data (20) (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 29). Here the instructor focuses on how the growth rate increases then decreases as the population approaches carrying capacity.
6. SYNTHESIS (~7 MINUTES)
To conclude, students are given the opportunity to synthesize the concepts covered throughout the lesson. The instructor summarizes how the growth rate differs in linear, exponential, and logistic growth curves (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 30). Then, the instructor provides a few examples of how the growth model can be applied, including economic, medical, and conservation predictions and decision-making (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 31). Students are presented with growth curves showing the population size over time for four different types of organisms (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slides 32-33).
TPSQ4. Describe the following populations over time:
In small groups, students discuss the population growth curves for bristlecone pine trees, grey wolves, bacteria, and red foxes. For each growth curve, they: 1) identify if density or abundance is shown; 2) describe what is happening to the population growth rate (increasing, decreasing, etc.); and 3) identify if the graph most closely resembles linear, exponential, logistic, or other growth. The goal is to allow students the time to synthesize all of the information gained throughout the lesson and to apply quantitative reasoning to different types of organisms.
After small group discussion, the instructor solicits answers from the class to determine how they answered each of the questions, focusing on the problem-solving techniques used. For example, the instructor can highlight looking at the y-axis to determine if abundance or density is measured, the importance of considering population size and rate of growth separately, and the role of carrying capacity in producing the logistic growth curve.
The instructor concludes the lesson by reviewing the learning outcomes and by answering any student questions (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 34).
TEACHING DISCUSSION
We used formative, real-time, and summative assessment to reflect on the effectiveness of this lesson. Here we discuss student responses to pre/post multiple-choice questions, exam questions, and a student attitudinal survey. Student responses to in-class questions are provided in the Progressing Through the Activity Section.
STUDENT PERFORMANCE AND CONCEPTUAL DIFFICULTIES
PRE/POST MULTIPLE-CHOICE QUESTIONS
Introductory biology students answered ten pre/post multiple-choice questions (abbreviated PPTQ for Pre/Post Test Questions). The questions can be grouped into four main categories that correspond with the parts of the lesson: 1) quantifying population size, 2) predicting population growth, 3) identifying and comparing growth rate, and 4) determining the influence of carrying capacity (Table 2). We report the percent correct for each question for those students who completed all components of the activity (n=433).
Across all three classes, the overall average pre-test score was 69% and the average post-test score was 78%. To calculate the normalized gain for overall scores on the pre/post-test, we used the following formula (31): (% of students who scored correct on the post-test - % of students who scored correct on the pre-test) / (100% - % of students who scored correct on the pre-test). The normalized gain for the pre/post-test is
We also calculated normalized gain scores (31) at the individual question level and found a range from
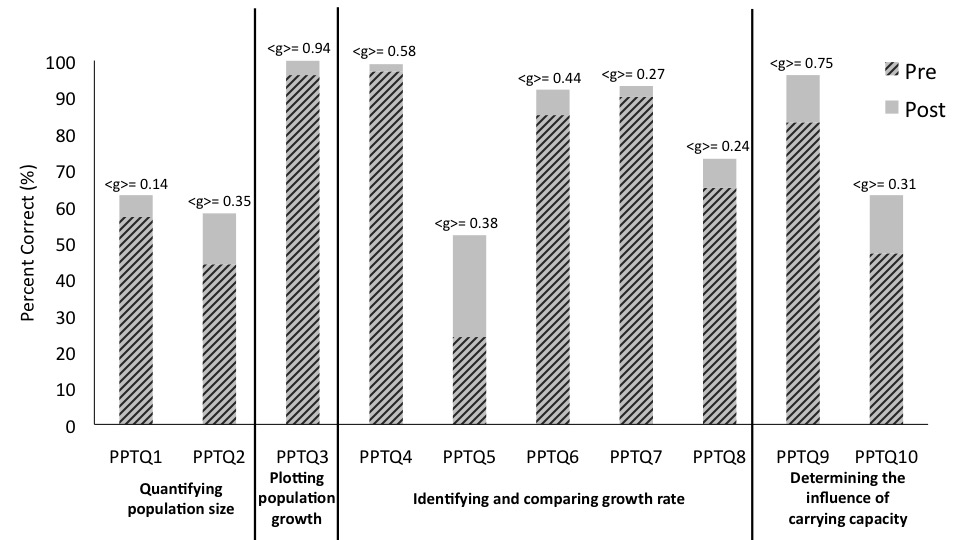
Figure 2. Student percent correct before and after instruction on pre/post-test questions (abbreviated PPTQ). Normalized gain <g> is provided for each question.
1. QUANTIFYING POPULATION SIZE
Two pre/post-test questions (PPTQ1 and PPTQ2) were designed to investigate students' ability to calculate density and abundance of a population (Table 2, Supporting File S4: Pre/Post-Test Questions and Student Responses). Experts would generally approach these questions by calculating density [density (D)= number of individuals (n) / unit volume (v)], and counting the number of individuals for abundance. After instruction, students showed improvement on both PPTQ1 and PPTQ2 (Figure 2).
All wrong answer choices for PPTQ1 and PPTQ2 targeted incorrect calculations and included incorrect units (Supporting File S4: Pre/Post-Test Questions and Student Responses). For PPTQ1, the density of the sunflowers is the same in two different quadrats even though they take up different areas (answer C), but students who missed this question on the post-test were roughly evenly divided between incorrect answer choice A (quadrat 1 has greater density, 19%) and answer choice B (quadrat 2 has greater density, 17%). For PPTQ2, the most common incorrect answer on the post-test was D (24%), where the density was incorrectly written as "5 individuals" and abundance was written as "20 individuals per square meter." These incorrect answers suggest that some students were still confused about what density measures and the correct units, and how density differs from abundance.
2. PLOTTING POPULATION GROWTH
One question on the pre/post-test (PPTQ3) asked students to plot data and select which of three given growth curves (linear, exponential, and logistic) their plot most resembles (Table 2, Supporting File S4: Pre/Post-Test Questions and Student Responses). Before and after instruction the percentage of students answering PPTQ3 correctly was high (Figure 2). This result suggests that students were able to successfully generate a plot when provided data and a graph with pre-made x-and-y axes.
3. IDENTIFYING AND COMPARING GROWTH RATE
Two pre/post-test questions (PPTQ4 and PPTQ5) asked students to determine how growth rate changes over time in exponential and logistic growth curves (Table 2, Supporting File S4: Pre/Post-Test Questions and Student Responses). Experts would generally approach these questions using the slope of the line. On the post-test, students were far more likely to predict the growth rate for an exponential growth model (PPTQ4, 99% correct) compared to a logistic growth model (PPTQ5, 52% correct) (Figure 2). The most common incorrect answer for PPTQ5 is that growth rate increases over time in a logistic model (incorrect answer A), where students likely equated a larger population size with a faster growth rate. This conceptual difficulty was previously reported for college students who were asked to compare speed at two different points along a graph; more than half of the students answered incorrectly, stating that the speed was fastest at the furthest distance on the graph, regardless of the slope (33,34).
For pre/post-test questions PPTQ6, PPTQ7, and PPTQ8 students compared growth rates within and between linear and exponential growth curves (Supporting File S4: Pre/Post-Test Questions and Student Responses). On the post-test, students performed well on a question that asked them to compare the growth rate between two points on a linear growth curve (PPTQ6, 92% correct) and on a question that asked them to compare two points on an exponential growth curve (PPTQ7, 93% correct). However, it was a more challenging for students to compare a point on a linear growth curve with a point on an exponential growth curve (PPTQ8, 73% correct). The most common incorrect answer is that that the growth rates were equal (answer C), which suggests that students are not consistently using the slope of the line to estimate growth rate.
4. DETERMINING THE INFLUENCE OF CARRYING CAPACITY
Two pre/post-test questions investigated students' ability to integrate the concept of carrying capacity in logistic growth curves (PPTQ9 and PPTQ10). For PPTQ9, students were asked whether carrying capacity impacts logistic growth curves (answer A, correct), exponential growth curves (answer B), both growth curves (answer C), or neither growth curve (answer D). The majority of students on the post-test (96%) answered this question correctly (answer A). However, in PPTQ10, where students were asked whether density-dependent growth impacts logistic growth curves (answer A, correct), exponential growth curves (answer B), both growth curves (answer C), or neither growth curve (answer D), only 63% of the students answered correctly. This result suggests that while students generally understand carrying capacity, they are more uncertain of the role of density-dependence.
EXAM QUESTIONS
Students were given four exam questions (abbreviated EQ) about population growth (Supporting File S5: Exam Questions and Student Responses; Figure 3). Students used the logistic model to calculate growth rate (EQ1), determine when growth rate would be fastest (EQ2, EQ3), and estimate carrying capacity (EQ4). For EQ1, 75% of students who completed the activity answered correctly while 55% of students who did not complete the activity provided the correct answer. 70% compared to 64% (completed activity/did not complete activity) answered correctly for EQ2, 97% compared to 96% for EQ3, and 99% compared to 98% for EQ4.
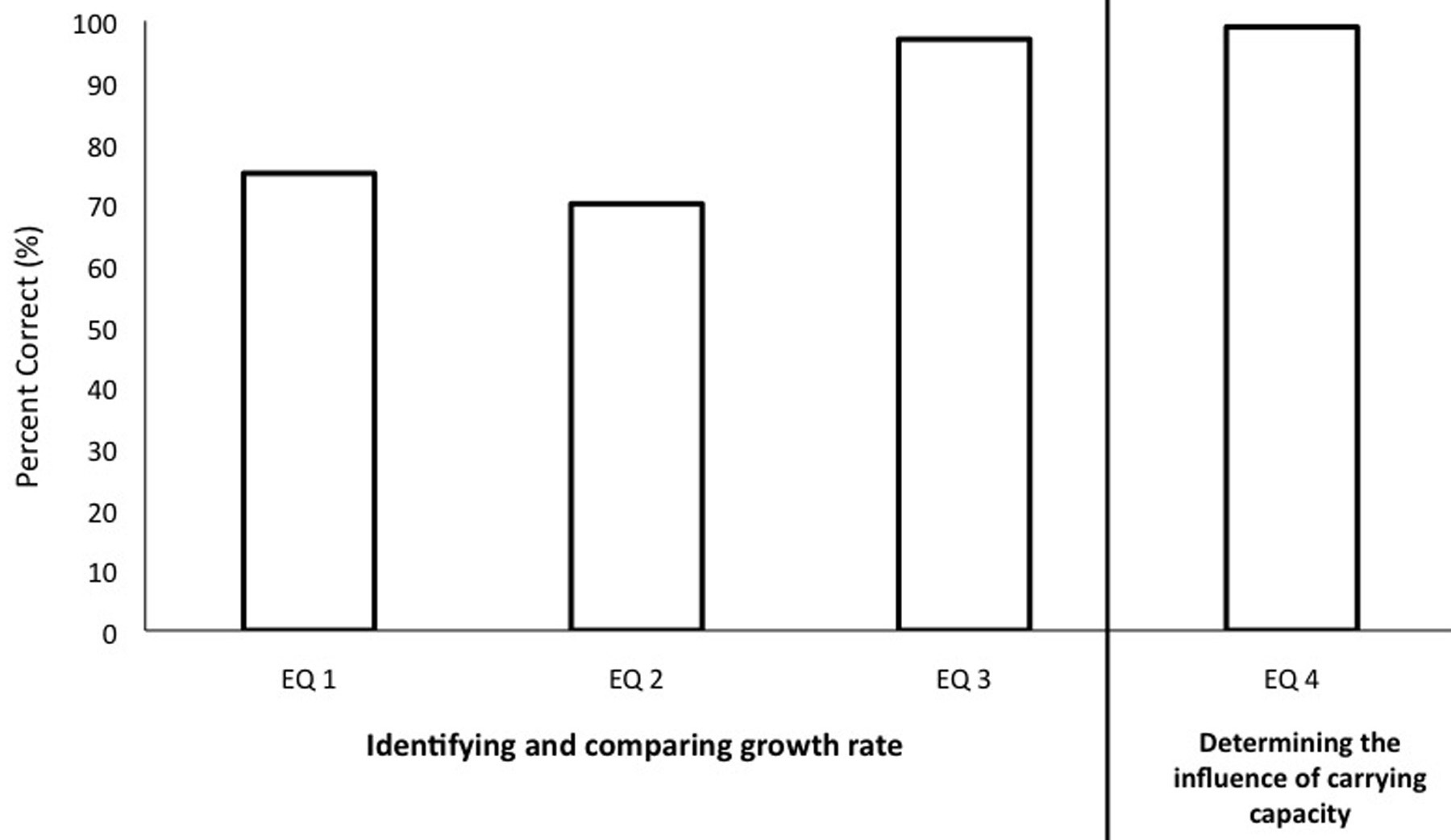
Figure 3. Student percent correct for exam questions (abbreviated EQ).
STUDENT PERCEPTIONS
Students were given a short survey on their perception of the lesson's usefulness immediately following the post-test (Supporting File S7: Attitudinal Survey Questions). Overall, students stated that participating in this lesson increased their understanding of how ecologists measure and model population size (88% agree/ strongly agree). When asked to explain, students provided reasons such as: "I learned about the different models of population growth and how to calculate growth rate for each;" "It gave me a clear understanding of how ecologists measure and model population size by giving me real-life examples and visuals to help me understand;" and "This lesson helped increase my understanding of how ecologists measure population size because it was hands on, fun, and engaging to do the calculations as an entire class."
The majority of students found the clicker questions, peer discussion, whole group discussion, and the in-class worksheet to be useful/very useful (Table 3). When asked to describe what parts of the population growth lesson were particularly useful to their learning, representative student comments included: "I found the peer discussion to be helpful because it helps me see different viewpoints that I would not have otherwise thought of;" "I really liked having the worksheet in the lesson because it made us more engaged with the problems in the lesson;" and "the clicker questions, class discussions, and worksheet all combined to help me better learn the material." Together, these results suggest that students enjoyed the lesson and perceived it as useful to their learning.
ADDITIONAL SUGGESTIONS TO ENHANCE STUDENT LEARNING WHILE USING THIS LESSON
Based on the student performance on the clicker, pre/post-test, and exam questions, we make the following recommendations to further improve student learning:
1. Density and abundance. Some students had difficulty calculating density, distinguishing between density and abundance, and identifying the correct units for density and abundance (Figure 2, PPTQ1 and PPTQ2). We recommend explicitly discussing the units of density and abundance as a valuable tool for students and providing more practice problems distinguishing between the two.
2. Calculations. A subset of students struggled in the lesson to understand and use equations to calculate growth rate for the three different growth curves. For example, it was our intention to use the suggested calculations that are part of CQ5 (Supporting File S1: Lesson Presentation Slides with Instructor Notes, slide 26) as an exercise to allow students to explore how changing the variables results in changes to the population model. Many students were unable to input values for the variables and their attention focused on this skill rather than conceptually understanding the equation. Students also requested more practice using the equations on a post-attitudinal survey (Supporting File S7: Attitudinal Survey Questions). There are a few online resources (e.g., 15) that students could complete as a homework assignment to increase familiarity with the variables.
3. Estimating growth rate. The pre/post-test revealed that students were more comfortable thinking about growth rates in exponential models than in logistic models. We also found that students could more easily make comparisons between points on the same growth curve than between points on different growth curves (Figure 2, PPTQ6-8). We therefore recommend emphasizing slope and additional opportunities for students to practice and complete problems where they estimate or calculate the slope in the context of population growth models.
4. Integrating density-dependent growth. Many students could not identify and explain how density influences the population models (Figure 2, PPTQ10). We think students would benefit from more time focused on density-dependence to help them connect the concepts of density-dependence, carrying capacity, and logistic growth.
CONCLUSIONS
This clicker-based lesson engages students in quantitative reasoning skills essential for population ecology: calculating and interpreting density and abundance; generating graphs from existing data; calculating growth rates from linear, exponential, and logistic growth curves; and making inferences about population growth over time using mathematical models. A diversity of inclusive teaching practices are used throughout the lesson, including clicker questions with the think-pair-share model, worksheets, and instructor led discussions. Assessment results reveal student learning and identify persistent areas of conceptual difficulty.
SUPPORTING MATERIALS
- S1. Population Growth Ecology-Lesson Presentation Slides with Instructor Notes.
- S2. Population Growth Ecology-Instructor Population Ecology Resources.
- S3. Population Growth Ecology-Clicker Questions and Student Responses.
- S4. Population Growth Ecology-Pre/Post-Test Questions and Student Responses.
- S5. Population Growth Ecology-Exam Questions and Student Responses.
- S6. Population Growth Ecology-Student Worksheet.
- S7. Population Growth Ecology-Attitudinal Survey Questions.
ACKNOWLEDGMENTS
We would like to thank Dr. Farahad Dastoor for allowing us to teach this lesson in his course. We would like to acknowledge the participation and contribution of all of the students who actively engaged in this lesson and answered our pre/post questions. Many others also contributed ideas and preliminary data to this project for which we are thankful. We would like to recognize Dr. Amanda Klemmer for inviting us to pilot the first version of this lesson in her course. The recommendations and suggestions of the University of Maine Biology and Ecology Department Teaching and Learning Journal Club, Maine Center for Research in STEM Education (RiSE Center), Smith lab, and the Ecology of Evolution and Everything Seminar (EES) were invaluable to the development of this lesson.
References
- National Research Council (NRC). 2003. BIO2010: Transforming undergraduate education for future research biologists. Washington, DC: National Academic Press.
- American Association for the Advancement of Science. 2009. Vision and change in undergraduate biology education: a call to action. Washington, DC: American Association for the Advancement of Science.
- Speth EB, Momsen JL, Moyerbrailean GA, Ebert-May D, Long TM, Wyse S, Linton D. 2010. 1, 2, 3, 4: infusing quantitative literacy into introductory biology. CBE Life Sci. Edu. 9:323-332.
- Hester S, Buxner S, Elfring L, Nagy L. 2014. Integrating quantitative thinking into an introductory biology course improves students' mathematical reasoning in biological contexts. CBE Life Sci. Edu. 13:54-64.
- Bravo A, Porzecanski A, Sterling E, Bynum N, Cawthorn M, Fernandez D. S, Vogler D. 2016. Teaching for higher levels of thinking: developing quantitative and analytical skills in environmental science courses. Ecosphere. 7:1-20.
- National Research Council (NRC). 2009. A new biology for the 21st century: Ensuring the United States leads the coming biology revolution. Washington, DC: National Academic Press.
- Picone C, Rhode J, Hyatt L, Parshall T. 2007. Assessing gains in undergraduate students' abilities to analyze graphical data. TIEE. 5:1-54.
- Kitchen E, Bell JD, Reeve S, Sudweeks RR, Bradshaw WS. 2003. Teaching cell biology in the large-enrollment classroom: methods to promote analytical thinking and assessment of their effectiveness. Cell Biol. Educ. 2:180-194.
- Urry LA, Cain ML, Wasserman SA, Minorsky PV, Jackson RB, Reece JB. 2014. Population Ecology and the Distribution of Organisms, p 818-844. In Biology in focus. Boston, MA:Pearson.
- Cotgreave P, Forseth I. 2002. Introducing biotic interactions and population models, p 94-107. In Introductory ecology. United Kingdom:Blackwell Science.
- Summers MM, Brownell S, Couch BA, Crowe A, Knight J, Semsar K, Wright CD, Smith, MK. submitted. EcoEvo-MAPS: An ecology and evolution assessment tool for introductory and advanced students.
- Munson BH. 1994. Ecological misconceptions. J. Environ. Edu. 25:30-34.
- D'Avanzo C. 2003. Research on learning: potential for improving ecology education. Front Ecol. Environ. 1:533-540.
- Hale BM, McCarthy ML. 2005. An introduction to population ecology. http://www.maa.org/press/periodicals/loci/joma/an-introduction-to-population-ecology. Accessed September 24, 2016.
- Jeanne R. 2003. Population dynamics. http://ats.doit.wisc.edu/biology/ec/pd/pd.html. Accessed September 24, 2016.
- Beckstead J, Lagasse AN, Robinson SR. 2011. Exploring the population dynamics of wintering bald eagles through long-term data. TIEE. 7:1-14.
- Aronhime B, Elderd BD, Wicks C, McMichael M, Eich E. 2013. Teaching exponential and logistic growth in a variety of classroom and laboratory settings. TIEE 9:1-26.
- Glase JC, Zimmerman M. 1993. Population ecology: experiments with protistans. In Beiswenger JM (ed), Experiments to teach ecology, vol 1. Washington, DC:ESA.
- Langin K, Sofaer H, Sillett S. 2009. Why study demography. http://hubbardbrookfoundation.org/migratory_birds/Pages/Background/Demography.html. Accessed September 24, 2016.
- Connell JH. 1961. Effects of competition, predation by Thia lapullus, and other factors on natural populations of the barnacle Balanus balanoides. Ecol. Monogr. 31:61-104.
- Smith MK, Jones FH, Gilbert SL, Wieman CE. 2013. The Classroom Observation Protocol for Undergraduate STEM (COPUS): a new instrument to characterize university STEM classroom practices. CBE Life Sci. Edu. 12:618-627.
- Smith MK, Vinson EL, Smith JA, Lewin JD, Stetzer M. 2014. A Campus-Wide Study of STEM Courses: New Perspectives on Teaching Practices and Perceptions. CBE Life Sci. Edu. 13:624-635.
- Armstrong MA. 2011. Small world: crafting an inclusive classroom (no matter what you teach). NEA Thought & Action Fall:51-61.
- Inclusive teaching strategies. 2016. https://www.cte.cornell.edu/teaching-ideas/building-inclusive-classrooms/inclusive-teaching-strategies.html. Accessed October 20, 2016.
- Martyn M. 2007. Clickers in the classroom: An active learning approach. Educause quarterly 30: 71.
- Crouch CH, Mazur E. 2001. Peer instruction: Ten years of experience and results. Am. J. Phys. 69:970-977.
- Smith MK, Wood WB, Adams WK, Wieman C, Knight JK, Guild N, Su TT. 2009. Why peer discussion improves student performance on in-class concept questions. Science 323:122-124.
- Smith MK, Wood WB, Krauter K, Knight JK. 2011. Combining peer discussion with instructor explanation increases student learning from in-class concept questions. CBE Life Sci. Edu. 10:55-63.
- Kothiyal A, Majumar R, Murthy S, Iyer S. 2013. Effect of think-pair-share in a large CS1 class: 83% sustained engagement. Proceedings of the 9(th) Annual ACM International Computing Education Research Conference, San Diego, CA.
- Lyman F. 1987. Think-Pair-Share: An expanding teaching technique. MAA-CIE Cooperative News. 1:1-2.
- Hake RR. 1998. Interactive-engagement versus traditional methods: a six-thousand-student survey of mechanics test data for introductory physics courses. Am. J. Phys. 66:64-74.
- Marx JD, Cummings K. 2007. Normalized change. Am. J. Phys. 75:87-91.
- McDermott L, Rosenquist M, Popp B, Van Zee E. 1983. Student difficulties in connecting graphs, concepts and physical phenomena. Presented at the American Educational Research Association meetings, Montreal, Canada.
- Mokros JR, Tinker RF. 1987. The impact of microcomputer-based labs on children's ability to interpret graphs. JRST. 24:369-383.
Article Files
Login to access supporting documents
An active-learning lesson that targets student understanding of population growth in ecology(PDF | 486 KB)
S1.Population Growth Ecology-Describing and modeling population sizes over time lesson presentation slides with instructor notes.pptx(PPTX | 5 MB)
S2.Population Growth Ecology-Instructor population ecology resources.docx(DOCX | 105 KB)
S3.Population Growth Ecology-Describing and modeling populations over time clicker questions and student responses.docx(DOCX | 127 KB)
S4.Population Growth Ecology-Describing and modeling populations over time pre-post questions and student responses.docx(DOCX | 329 KB)
S5.Population Growth Ecology-Describing and modeling populations over time exam questions and student responses.docx(DOCX | 171 KB)
S6.Population Growth Ecology-Describing and modeling populations over time student lecture worksheet.pdf(PDF | 215 KB)
S7.Population Growth Ecology-Describing and modeling populations over time attitudinal survey questions.docx(DOCX | 79 KB)
- License terms

Comments
Comments
0 Like
Alfonso Pineda @ on
Thanks for this great teaching resource.
Alfonso
Copy link Report abuse